
- #Fundamental theorem of calculus calculator how to#
- #Fundamental theorem of calculus calculator free#
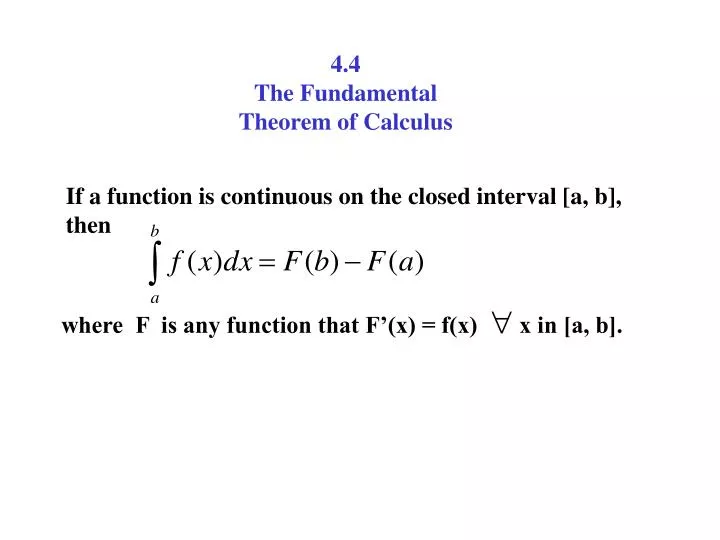
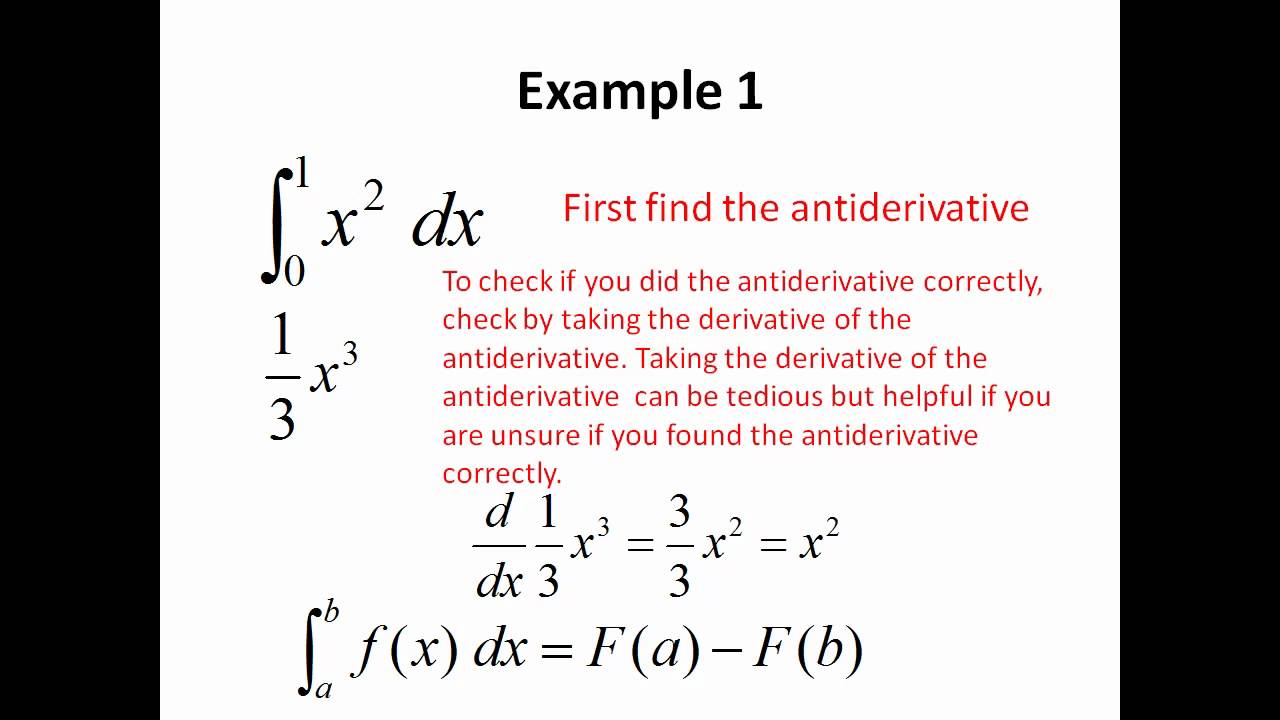
First, it states that the indefinite integral of a function can be reversed by differentiation, \int_a^b f(t)\, dt = F(b)-F(a). Therefore, since F F is the antiderivative of. Check out how this page has evolved in the past. As we talked about in lecture, the Fundamental Theorem of Calculus shows the relationship between derivatives and integration and states that if f is the derivative of another function F F then, b a f (x)dx a b f ( x) d x F (b)F (a) F ( b) F ( a). We will now look at the second part to the Fundamental Theorem of Calculus which gives us a method for evaluating definite integrals without going through the tedium of evaluating limits.
#Fundamental theorem of calculus calculator how to#
The Fundamental Theorem tells us how to compute the derivative of functions of the form R x a f(t) dt. The second fundamental theorem of calculus holds for f a continuous function on an open interval I and a any point in I, and states that if F is defined by the integral (antiderivative) F(x)=int_a^xf(t)dt, then F^'(x)=f(x) at each point in I, where F^'(x) is the derivative of F(x). – differential calculus and integral calculus. Fundamental Theorem of Calculus says that differentiation and … Pick any function f(x) 1. How Part 1 of the Fundamental Theorem of Calculus defines the integral.
#Fundamental theorem of calculus calculator free#
Type in any integral to get the solution, free steps and graph Once again, we will apply part 1 of the Fundamental Theorem of Calculus. This theorem gives the integral the importance it has. As mentioned earlier, the Fundamental Theorem of Calculus is an extremely powerful theorem that establishes the relationship between differentiation and integration, and gives us a way to evaluate definite integrals without using Riemann sums or calculating areas. This part describes how to evaluate definite integrals without having to calculate limits of Riemann sums. Fundamental Theorem, Part 2 (The Evaluation Theorem) We now come to the second part of the Fundamental Theorem of Calculus. The fundamental theorem of calculus has two parts. Then Theorem 1 in Section 3.1 shows that F is continuous for every point of a, b.

The second part states that the indefinite integral of a function can be used to calculate any definite integral, \intab f(x)\,dx F(b) - F(a). First, it states that the indefinite integral of a function can be reversed by differentiation, \intab f(t)\, dt F(b)-F(a). View wiki source for this page without editing. The fundamental theorem of calculus has two separate parts. The fundamental theorem of calculus has two separate parts.


 0 kommentar(er)
0 kommentar(er)
